Coffee to Theorems

Marilyn Burns (@mburnsmath) recently blogged about a problem she came across via NCTM’s Teaching Children Mathematics and Mike Flynn

 

I’m looking for some Ts to try out my @TCM_at_NCTM task w/ their students & share their work. Thanks! #ElemMathChat pic.twitter.com/4ytSf9DtAS

— Mike Flynn (@MikeFlynn55) August 15, 2016

This Bike Shop Lesson ended up being rich not just for the students, but also for herself. In exploring the underlying structure, you can touch on many different topics. See Marilyn’s blog, Henri Picciotto’s blog, and Simon Gregg’s tweets for a more there!

 

---

As I read these thoughts from others it reminded me of a similar lesson I did with high school Algebra 2 students. It also dealt with partitions of numbers, but exploring different constraints than the unicycles, bicycles, and tricycles. In exploring those constraints, my students found some interesting patterns including Pascal’s Triangle, powers of two, the Fibonacci sequence,

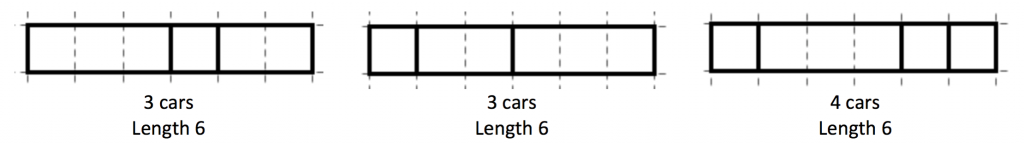
Traincar Number Lesson

Here are three trains with length 6, but they are made up of different numbers of cars and types of cars. How many different trains are there?

I purposely left the question vague because I wanted students to interpret it in a variety of ways. Influenced by Yackel and Cobb’s 1996 article, “Sociomathematical Norms,” I wanted students to develop their own agreements in their groups about what counted as different.

[note: I did explicitly ask my students about trains of length 5 to start them off. I may choose otherwise in the future]

Students refine the question

I gave the students cutouts (included in PDF) to support a few aspects of problem solving. First, the scaled cutouts support non-numeric representations. Second, the easy-to-change nature means students can re-arrange the blocks quickly and are not locked to what they have first represented. Third, they must formalize thinking from the cutouts to more permanent representations. And finally, the cutouts are easily worked by more than one person so it can promote discussion in a group.

Here are some of the thoughts my students had:

1. Is 2+2+1 different than 2+1+2 ? What if they’re the same?
2. What if you don’t allow copies of a car? (so 2+2+1 is not allowed because there are two “2s”)
3. Are all “1 cars” the same?
4. Can we use negative cars? (like a car of length “-2” for example)
5. Can we use partial cars? (“1.5”)
6. Are reflected trains the same? (2+2+1 and 1+2+2 might be the same, while 2+1+2 is different)
7. what if we are limited by how many cars? (only allowed to use exactly three cars, for example)

I was very impressed by how much the students embraced the chance to question the rules and develop their own. Its something we teachers should do more often! I expected to see questions 1 and 6 from the students, but the other questions each surprised me in some way. I learned a lot about what my students could do when given the chance. They revealed a lot of thoughts they had about permutations, combinations, sorting, number, structure… I was able to grab onto this knowledge later in the year as we formally talked about those subjects.

The freedom of exploration in the problem let students from multiple ability levels contribute. One student who was struggling was the one asking about negative car sizes. Its the kind of question that makes you pause; you may wonder if he’s asking about this does he understand the problem? I am very glad I let him explore it with his group. The answer, “infinite number of trains!” is much more meaningful when the student discovers it, rather than the teacher disallowing the investigation by providing the answer.

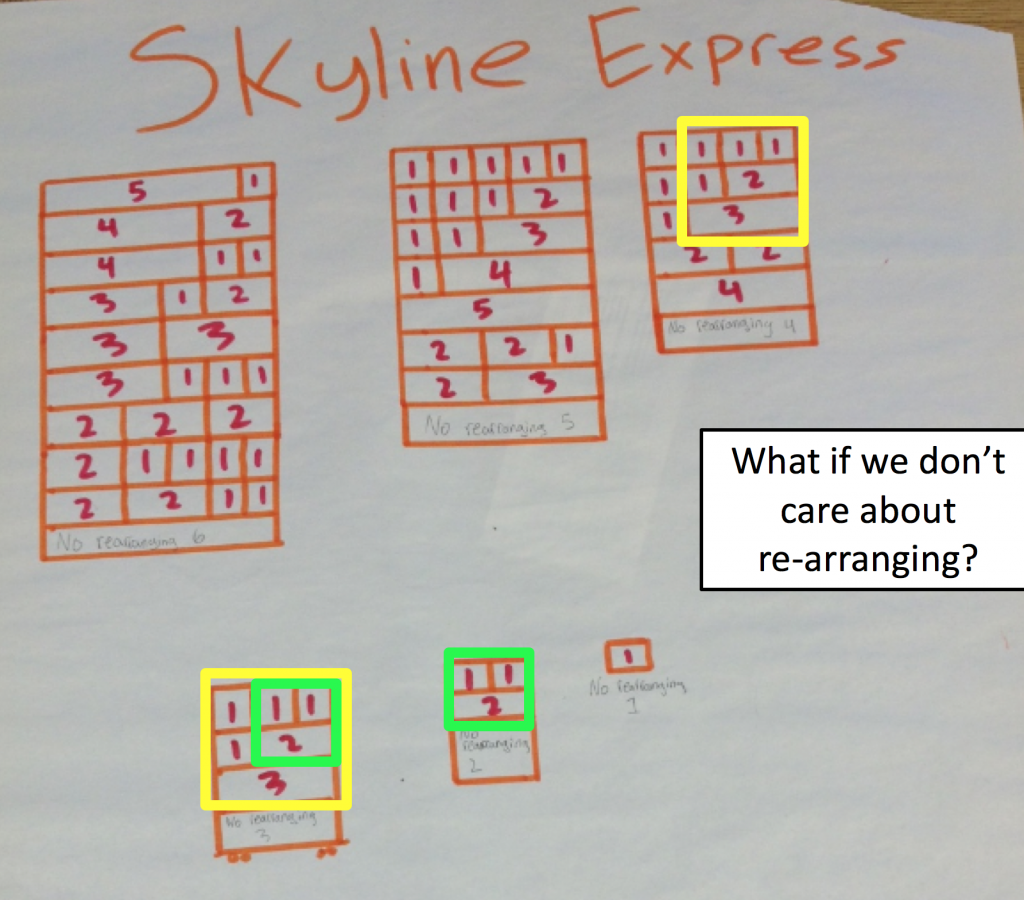
Student work

Also, I was able to let the students experience the results of testing their own conjectures. Here are some results of their investigations: (there are some small errors in their work)

1	3
4	7

Most groups found the pattern that with certain assumptions, the number of trains of length N is 2^N. But the exposure to other assumptions generating other patterns was a great place for a meta-discussion about mathematical practice, and the hidden structure of numbers.

We discussed how some groups patterns fit together and how as a class we explored a lot of boundaries to the problem. We talked then about some of the boundaries we did not explore: such as limitations to only certain lengths of cars. (Notice the limitation to 1s, 2s, and 3s cars is the Cycling Shop Problem!

So when Marilyn and Henri were sharing ideas about the cycling shop problem I recalled my students work and how we were linking the various assumptions made into a larger structure. I played with thinking about the unicycles, bicycles and tricycles built from 8 wheels inside the organization of combinations and partitions.

How fun was it to come back to this problem myself and dig around for new concepts! And its not nearly done…

Limiting to only 1s and 2s has another fun result that I wont spoil here… try it yourself! try it with your students!) Another extension is to limit to prime length cars only. I concluded with sharing the Goldbach Conjecture:

Every even integer greater than 2 can be expressed as the sum of two primes

I asked my students what they thought, how they might approach it, how its connected to the traincars they’ve thought about. Then I let them know that the problem is unsolved. “You are part of the community of mathematicians… testing assumptions, making conjectures, organizing and sharing ideas.” It was one of my favorite lessons I’ve done. (Also a great way to lead into winter break… “Your HW for break is to solve Goldbach, have fun!”)

Traincar Lesson PDF – this is ‘localized’ with a playful name for my school and timing in the year (“Skyline Express”) but also I gave it as a participation quiz, so some of the introduction of the task in this pdf is specific to that classroom format.

Differentiation to all levels

Notice aspects of these partition problems have piqued curiosity of students from elementary school, to high school, to teachers, and of course to professional mathematicians. When the learner has a chance to refine the question for themselves and explore their own ideas, the task is becomes personalized. And when the tasks are personalized then we can make them socialized: people sharing their own ideas and work.

---

References

Skyline Express Lesson Materials PDF – Scott Farrar 2013

NCTM – Teaching Children Mathematics – The Cycling Shop http://www.nctm.org/Publications/Teaching-Children-Mathematics/2016/Vol23/Issue1/The-cycling-shop/ August 2016

Yackel, E., & Cobb, P. (1996). Sociomathematical Norms, Argumentation, and Autonomy in Mathematics.Journal for Research in Mathematics Education, 27(4), 458-477. doi:1. Retrieved from http://www.jstor.org/stable/749877 doi:1